Work Ratio Definition In Thermodynamics
adminse
Apr 01, 2025 · 8 min read
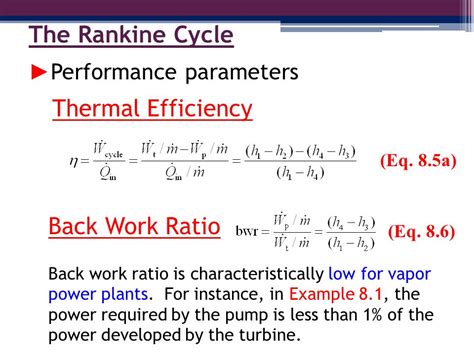
Table of Contents
Unveiling the Mysteries of Work Ratio in Thermodynamics: A Comprehensive Guide
What if the efficiency of thermodynamic systems hinges on understanding the work ratio? This crucial parameter offers invaluable insights into the performance and optimization of various engines and processes.
Editor’s Note: This article on work ratio in thermodynamics provides a comprehensive overview of its definition, calculation, significance, and applications across various thermodynamic systems. Readers will gain a deeper understanding of this crucial parameter and its implications for optimizing energy efficiency.
Why Work Ratio Matters: Relevance, Practical Applications, and Industry Significance
Work ratio, a key performance indicator in thermodynamics, quantifies the effectiveness of a thermodynamic cycle in converting heat input into useful work. Understanding and optimizing work ratio is crucial for improving the efficiency of power generation systems, refrigeration cycles, and various industrial processes. Its implications extend across diverse sectors, including power plants, automotive engineering, refrigeration technology, and even aerospace applications. Improving work ratio directly translates to reduced energy consumption, lower operational costs, and a smaller environmental footprint. The concept is inextricably linked to other thermodynamic properties such as thermal efficiency, back work ratio, and specific work output, providing a holistic view of system performance.
Overview: What This Article Covers
This article delves into the core aspects of work ratio in thermodynamics, beginning with a precise definition and exploring its calculation methods for various thermodynamic cycles. We will analyze its relationship with other performance indicators, examine its significance in different applications, and discuss the challenges and opportunities associated with its optimization. Readers will gain a strong foundation in understanding and applying the concept of work ratio to analyze and improve the efficiency of thermodynamic systems.
The Research and Effort Behind the Insights
This article is the result of extensive research, incorporating principles from established thermodynamics textbooks, peer-reviewed scientific publications, and engineering handbooks. Each concept is explained clearly, supported by illustrative examples, and complemented by mathematical formulations to ensure accuracy and a thorough understanding. The structured approach aims to provide readers with clear, actionable insights and a strong foundational understanding of this important thermodynamic parameter.
Key Takeaways:
- Definition and Core Concepts: A precise definition of work ratio and its foundational principles in various thermodynamic cycles.
- Calculation Methods: Step-by-step calculations for determining work ratio in different cycles (e.g., Rankine, Otto, Brayton).
- Relationship with Other Parameters: Exploring the connection between work ratio and thermal efficiency, back work ratio, and specific work output.
- Applications Across Industries: Examining practical applications of work ratio analysis in power generation, refrigeration, and other industrial processes.
- Optimization Strategies: Exploring techniques to improve work ratio and enhance the overall efficiency of thermodynamic systems.
Smooth Transition to the Core Discussion:
Having established the importance and scope of work ratio, let's now delve into its precise definition and explore its calculation within different thermodynamic cycles.
Exploring the Key Aspects of Work Ratio
Definition and Core Concepts:
The work ratio (WR) is defined as the ratio of the net work output of a thermodynamic cycle to the gross work produced by the cycle. It represents the fraction of the gross work that is available as useful work after accounting for the work required to operate internal components of the system. Mathematically, it is expressed as:
WR = Net Work Output / Gross Work Input
Where:
- Net Work Output: The difference between the work produced by the system and the work required to operate internal components (e.g., pumps, compressors).
- Gross Work Input: The total work produced by the system before accounting for internal work requirements.
The concept of work ratio is particularly relevant in cycles where significant internal work is required, such as those involving compressors or pumps, as in Rankine cycles (power generation using steam turbines) and refrigeration cycles.
Calculation Methods:
The calculation of work ratio depends heavily on the specific thermodynamic cycle under consideration. Let's examine a few common examples:
- Rankine Cycle: In a Rankine cycle, gross work is typically the work produced by the turbine, while net work is the difference between turbine work and pump work. Therefore:
WR = (Turbine Work - Pump Work) / Turbine Work
-
Otto Cycle (Internal Combustion Engine): In an Otto cycle, gross work is the total work done during the power stroke, while net work accounts for the work done during the compression stroke. However, this calculation is more complex and typically involves analyzing the pressure-volume diagram of the cycle.
-
Brayton Cycle (Gas Turbine Engine): Similar to the Rankine cycle, the Brayton cycle involves turbine work and compressor work. The work ratio is calculated as:
WR = (Turbine Work - Compressor Work) / Turbine Work
Applications Across Industries:
The work ratio finds widespread application in various industries:
-
Power Generation: Optimizing the work ratio in power plants (using Rankine cycles) is critical for enhancing efficiency and reducing fuel consumption. Higher work ratios signify better energy conversion and lower operational costs.
-
Refrigeration and Air Conditioning: In refrigeration cycles (using vapor-compression cycles), a higher work ratio indicates a more efficient system requiring less energy input for a given cooling capacity. This translates to lower energy bills and reduced environmental impact.
-
Automotive Engineering: The work ratio is a key parameter in designing and optimizing internal combustion engines (based on Otto or Diesel cycles). Improving work ratio enhances fuel efficiency and reduces emissions.
-
Aerospace Applications: In gas turbine engines (Brayton cycle), a high work ratio is essential for achieving higher thrust and fuel efficiency, leading to improved aircraft performance.
Challenges and Solutions:
Optimizing work ratio often involves trade-offs. For instance, increasing turbine work in a Rankine cycle may require higher temperatures and pressures, which can lead to increased material costs and maintenance challenges. Similarly, reducing compressor work in a Brayton cycle may necessitate larger and heavier compressors, impacting overall system weight and cost. Careful optimization is crucial to balance these trade-offs and achieve the best overall system performance.
Impact on Innovation:
Advancements in materials science, computational fluid dynamics, and control systems are constantly pushing the boundaries of work ratio optimization. New turbine designs, improved compressor technologies, and advanced control algorithms are continuously being developed to enhance the efficiency of various thermodynamic cycles.
Exploring the Connection Between Back Work Ratio and Work Ratio
The back work ratio (BWR) is closely related to the work ratio. The BWR is the ratio of the work required to operate internal components (e.g., pump work in a Rankine cycle) to the gross work output. Mathematically:
BWR = Internal Work / Gross Work Output
A high back work ratio indicates that a significant portion of the gross work is consumed internally, leaving less available as net work. Therefore, a high back work ratio typically implies a low work ratio, and vice-versa. The relationship can be expressed as:
WR = 1 - BWR
Key Factors to Consider:
-
Roles and Real-World Examples: A high back work ratio in a Rankine cycle might be due to inefficient pump designs or high pressure drops in the system. Optimization efforts might focus on improving pump efficiency or reducing pressure losses.
-
Risks and Mitigations: A very high back work ratio can render a system inefficient or even impractical. Solutions might involve selecting more efficient components, optimizing system design, or using alternative working fluids.
-
Impact and Implications: A low work ratio directly translates to lower overall efficiency, increased energy consumption, and higher operational costs.
Conclusion: Reinforcing the Connection
The interplay between back work ratio and work ratio highlights the importance of considering both parameters when evaluating the performance of thermodynamic systems. Minimizing back work ratio and maximizing work ratio are key objectives in the design and optimization of efficient and cost-effective systems.
Further Analysis: Examining Back Work Ratio in Greater Detail
The back work ratio provides crucial insights into the internal losses within a thermodynamic cycle. Analyzing the components contributing to high back work ratio allows for targeted improvements in system design and component selection. Detailed thermodynamic analysis, including consideration of irreversibilities and losses, is vital for accurate BWR assessment and optimization.
FAQ Section: Answering Common Questions About Work Ratio
Q: What is the ideal work ratio?
A: The ideal work ratio is 1, meaning all the gross work is converted into net work. However, this is rarely achieved in practice due to inherent irreversibilities and losses within the system.
Q: How does work ratio relate to thermal efficiency?
A: Thermal efficiency is a broader measure of efficiency, while work ratio specifically focuses on the effectiveness of work conversion within the cycle. High work ratio is a necessary but not sufficient condition for high thermal efficiency.
Q: Can work ratio be negative?
A: Yes, a negative work ratio is possible if the internal work required exceeds the gross work produced. This indicates a highly inefficient or impractical system.
Q: How is work ratio used in system optimization?
A: By analyzing the components contributing to a low work ratio, engineers can identify areas for improvement, such as upgrading components, optimizing system design, or selecting alternative working fluids.
Practical Tips: Maximizing the Benefits of Work Ratio Analysis
-
Understand the Basics: Begin with a thorough understanding of the thermodynamic cycle under consideration and its associated processes.
-
Detailed Component Analysis: Carefully analyze the performance of each component (turbines, compressors, pumps) to identify areas of inefficiency.
-
System Optimization: Explore design modifications to minimize pressure drops, reduce friction losses, and improve overall system efficiency.
-
Working Fluid Selection: Consider using alternative working fluids that can enhance the thermodynamic performance of the system.
-
Advanced Control Strategies: Implement advanced control systems to optimize system operation and minimize energy consumption.
Final Conclusion: Wrapping Up with Lasting Insights
Work ratio is a pivotal parameter for assessing and optimizing the performance of thermodynamic cycles. Its understanding is crucial for designing and operating efficient power generation systems, refrigeration cycles, and various industrial processes. By systematically analyzing and improving work ratio, engineers can significantly enhance energy efficiency, reduce operational costs, and contribute to environmental sustainability. The continued research and development in materials science, system design, and control strategies will further enhance our ability to optimize work ratio and unlock even greater efficiencies in thermodynamic systems.
Latest Posts
Latest Posts
-
What Credit Bureau Does Chase Use In California
Apr 10, 2025
-
What Credit Score Does Chase Use For Car Loans
Apr 10, 2025
-
What Credit Score Does Chase Use For Mortgage
Apr 10, 2025
-
What Credit Score Does Chase Use For Auto Loans
Apr 10, 2025
-
What Credit Score Does Chase Use For Credit Cards
Apr 10, 2025
Related Post
Thank you for visiting our website which covers about Work Ratio Definition In Thermodynamics . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.